Derive lens formula for convex lens.
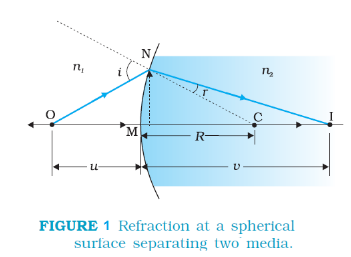
Figure 1 shows the geometry of formation of image I of an object O on the principal axis of a spherical surface with center of curvature C, and radius of curvature R. The rays are incident from a medium of refractive index n1, to another of refractive index n2.
As before, we take the aperture (or the lateral size) of the surface to be small compared to other distances involved, so that small angle approximation can be made. In particular, NM will be taken to be nearly equal to the length of the perpendicular from the point N
on the principal axis. We have, for small angles,
![]()
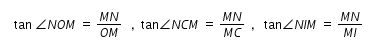
Now for ΔNOC, i is exterior angle. Therefore i = ∠NOM +∠NCM
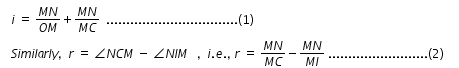
By Snell’s law, n1 sin i = n2 sin r or for small angles , n1 i = n2 r …(3)
substituting for i and r from (1) and (2), we simplify and rewrite eqn.(3) as
![]()
Equation (4) gives us a relation between object and image distance in terms of refractive index of the medium and the radius of curvature of the curved spherical surface. It holds for any curved spherical surface.
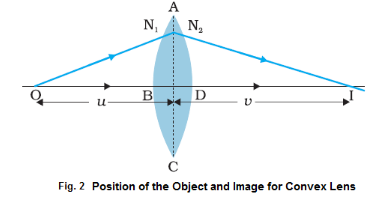
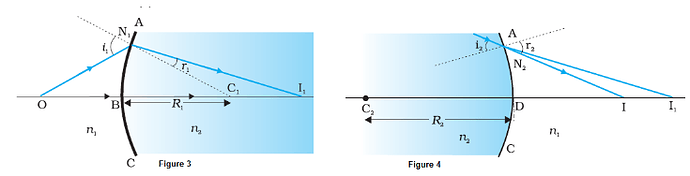
Figure 2 shows the geometry of image formation by a double convex lens. The image formation can be seen in terms of two steps:
(i) The first refracting surface forms the image I1 of the object O [ figure. 3]. The image I1 acts as a virtual object for the second surface
that forms the image at I [ figure 4. ]. Applying Eq. ( 4 ) to the first interface ABC, we get
A similar procedure applied to the second interface ADC gives,
For a thin lens, BI1 = DI1. Adding Eqs. (5) and (6), we get
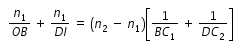
Suppose the object is at infinity, i.e., OB → ∞ and DI = f, Eq. (6 ) gives
The point where image of an object placed at infinity is formed is called the focus F, of the lens and the distance f gives
its focal length. A lens has two foci, F and F′, on either side of it . By the sign convention, BC1 = + R1, DC2 = –R2
So Eq. (7) can be written as
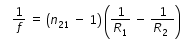 …(8)
…(8)because n21 = n2 / n1 .Equation (8) is known as the lens maker’s formula. It relates the focal length of given lens to the
radii of curvature of lens and also the refractive index of material of lens. It is useful to design lenses of desired focal length
using surfaces of suitable radii of curvature. Note that the formula is true for a concave lens also. In that case R1 is negative,
R2 positive and therefore, f is negative.
From Eqs. (5 ) and (6), we get
![]() …(9)
…(9)
Again, in the thin lens approximation, B and D are both close to the optical centre of the lens. Applying the sign convention,
BO = – u, DI = +v, we get
![]() …(10)
…(10)
Equation (10) is the familiar thin lens formula. Though we derived it for a real image formed by a convex lens, the formula is valid for both
convex as well as concave lenses and for both real and virtual images.