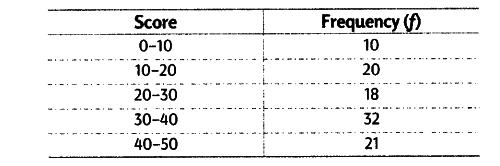
Compute mode from the following data
By observation method, it is clear that the modal value lies in the group of 30-40 because it has the highest frequency.
$ l_{ 1 }$ = 30, $ f_{ 0 }$ = 18, $ f_{ 1 }$ = 32, $ f_{ 2 }$ = 21 and c = 10
Now, Mode = $ l_{ 1 }$ + $ f_{ 1 }$ - $ f_{ 0 }$ / 2 $ f_{ 1 }$ - $ f_{ 0 }$ - $ f_{ 2 }$ X c = 30 + 32-18/2x32-18-21 X 10
= 30 +14/25 X10 = 30+5.6 = 35.6
Hence, the modal value is 35.6 score.