PS and RT are medians of ∆ PQR and SM | | RT. Prove that QM=1/4 PQ

CBSE Class 9 CBSE Class 9 Maths
1

In the following figure, PS and RT are medians of ∆ PQR and SM | | RT. Prove that
QM=1/4 PQ.
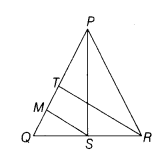
image

2

Given PS and RT are the medians of ∆ PQR, i.e. S and T are the mid-points of QR and PQ respectively,
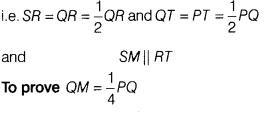
image
Proof In ∆ QTR, S is the mid-point of QR and SM || RT So, by converse of mid-point theorem, M is mid-point of QT
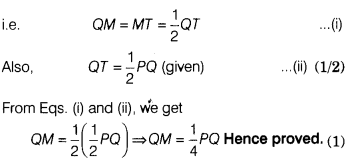image .