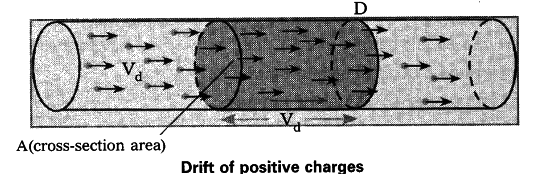
- Consider a conductor with cross-sectional area A. Assume that the two ends of the conductor are connected to a battery to make the current flow through it.
- Let ‘{{V}_{d}}’ be the drift speed of the charges and ‘n’ be the number of charges present in the conductor in an unit volume.
- The distance covered by each charge in one second is ‘{{V}_{d}}’.
- Then the volume of the conductor for this distance = A{{V}_{d}}
- The number of charges contained in that volume = n.A{{V}_{d}}
- Let q be the charge of each carrier.
- Then the total charges crossing the cross-sectional area at position D in one second is 'nq A{{V}_{d}}'.
This is equal to electric current.
.-. Electric current I = nq A{{V}_{d}}.
Therefore , {{V}_{d}} = 1/nqA